Truncated infinite-order triangular tiling
In geometry, the truncated infinite-order triangular tiling is a uniform tiling of the hyperbolic plane with a Schläfli symbol of t{3,∞}.
| Infinite-order truncated triangular tiling | |
|---|---|
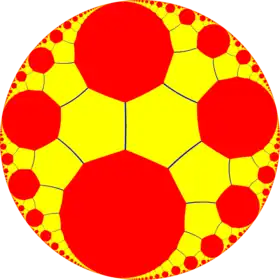 Poincaré disk model of the hyperbolic plane | |
| Type | Hyperbolic uniform tiling |
| Vertex configuration | ∞.6.6 |
| Schläfli symbol | t{3,∞} |
| Wythoff symbol | 2 ∞ | 3 |
| Coxeter diagram | |
| Symmetry group | [∞,3], (*∞32) |
| Dual | apeirokis apeirogonal tiling |
| Properties | Vertex-transitive |
Symmetry
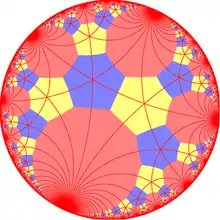
Truncated infinite-order triangular tiling with mirror lines, 

 .
.
The dual of this tiling represents the fundamental domains of *∞33 symmetry. There are no mirror removal subgroups of [(∞,3,3)], but this symmetry group can be doubled to ∞32 symmetry by adding a mirror.
| Type | Reflectional | Rotational |
|---|---|---|
| Index | 1 | 2 |
| Diagram |  |
 |
| Coxeter (orbifold) |
[(∞,3,3)] (*∞33) |
[(∞,3,3)]+ (∞33) |
Related polyhedra and tiling
This hyperbolic tiling is topologically related as a part of sequence of uniform truncated polyhedra with vertex configurations (6.n.n), and [n,3] Coxeter group symmetry.
| *n32 symmetry mutation of truncated tilings: n.6.6 | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sym. *n42 [n,3] |
Spherical | Euclid. | Compact | Parac. | Noncompact hyperbolic | |||||||
| *232 [2,3] |
*332 [3,3] |
*432 [4,3] |
*532 [5,3] |
*632 [6,3] |
*732 [7,3] |
*832 [8,3]... |
*∞32 [∞,3] |
[12i,3] | [9i,3] | [6i,3] | ||
| Truncated figures |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 | |
| Config. | 2.6.6 | 3.6.6 | 4.6.6 | 5.6.6 | 6.6.6 | 7.6.6 | 8.6.6 | ∞.6.6 | 12i.6.6 | 9i.6.6 | 6i.6.6 | |
| n-kis figures |
 |
 |
 |
 |
 |
 |
 |
|||||
| Config. | V2.6.6 | V3.6.6 | V4.6.6 | V5.6.6 | V6.6.6 | V7.6.6 | V8.6.6 | V∞.6.6 | V12i.6.6 | V9i.6.6 | V6i.6.6 | |
| Paracompact uniform tilings in [∞,3] family | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Symmetry: [∞,3], (*∞32) | [∞,3]+ (∞32) |
[1+,∞,3] (*∞33) |
[∞,3+] (3*∞) | |||||||
= |
= |
= |
= | |||||||
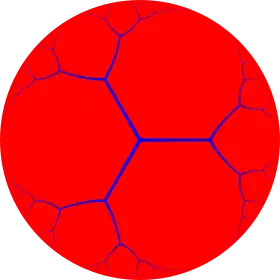 |
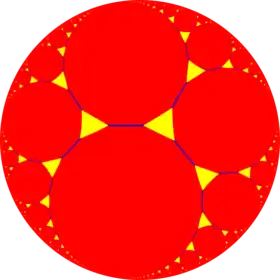 |
 |
 |
 |
 |
 |
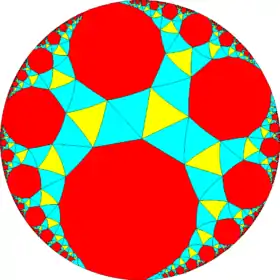 |
 |
 | |
| {∞,3} | t{∞,3} | r{∞,3} | t{3,∞} | {3,∞} | rr{∞,3} | tr{∞,3} | sr{∞,3} | h{∞,3} | h2{∞,3} | s{3,∞} |
| Uniform duals | ||||||||||
 |
 |
 |
 |
 |
 |
 |
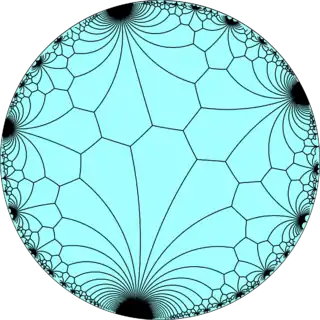 |
 |
||
| V∞3 | V3.∞.∞ | V(3.∞)2 | V6.6.∞ | V3∞ | V4.3.4.∞ | V4.6.∞ | V3.3.3.3.∞ | V(3.∞)3 | V3.3.3.3.3.∞ | |
| Paracompact hyperbolic uniform tilings in [(∞,3,3)] family | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Symmetry: [(∞,3,3)], (*∞33) | [(∞,3,3)]+, (∞33) | ||||||||||
 |
 |
 |
 |
 |
 |
 |
 | ||||
| (∞,∞,3) | t0,1(∞,3,3) | t1(∞,3,3) | t1,2(∞,3,3) | t2(∞,3,3) | t0,2(∞,3,3) | t0,1,2(∞,3,3) | s(∞,3,3) | ||||
| Dual tilings | |||||||||||
 |
 |
||||||||||
| V(3.∞)3 | V3.∞.3.∞ | V(3.∞)3 | V3.6.∞.6 | V(3.3)∞ | V3.6.∞.6 | V6.6.∞ | V3.3.3.3.3.∞ | ||||
See also
Wikimedia Commons has media related to Uniform tiling 6-6-i.
References
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
- "Chapter 10: Regular honeycombs in hyperbolic space". The Beauty of Geometry: Twelve Essays. Dover Publications. 1999. ISBN 0-486-40919-8. LCCN 99035678.
External links
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.

