Van der Meer formula

The Van der Meer formula is a formula for calculating the required stone weight for armourstone under the influence of (wind) waves. This is necessary for the design of breakwaters and shoreline protection. Around 1985 it was found that the Hudson formula in use at that time had considerable limitations (only valid for permeable breakwaters and steep (storm) waves). That is why the Dutch government agency Rijkswaterstaat commissioned Deltares to start research for a more complete formula. This research, conducted by Jentsje van der Meer, resulted in the Van der Meer formula in 1988, as described in his dissertation.[1] This formula reads [2] [3]

{\frac {H_{s}}{\Delta \cdot d_{n50}}}={\begin{cases}c_{p}P^{0.18}\left({\frac {S}{\sqrt {N}}}\right)^{0.2}\xi _{m}^{-0.5}&{\mbox{when }}\xi _{m}<\xi {cr}\quad [plunging]\\c_{s}P^{-0.13}\left({\frac {S}{\sqrt {N}}}\right)^{0.2}\xi _{m}^{P}{\sqrt {cot\alpha }}&{\mbox{when }}\xi _{m}\geq \xi {cr}\quad [surging]\end{cases}}

and

\xi _{cr}=\left[{\frac {c_{p}}{c_{s}}}P^{0.31}{\sqrt {tan\alpha }}\right]^{\frac {1}{P+0.5}}

In this formula:

H_s = Significant wave height at the toe of the construction

Δ = relative density of the stone (= (ρ_s -ρ_w)/ρ_w) where ρ_s is the density of the stone and ρ_w is the density of the water

d_n50 = nominal stone diameter

α = breakwater slope

P = notional permeability

S = Damage number

N = number of waves in the storm

ξ_m = the Iribarren number calculated with the Tm

For design purposes, for the coefficient c_p the value of 5,2 and for c_s the value 0,87 is recommended.[2]

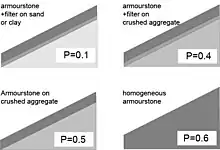
Notional permeability according to Van der Meer (1988)

The value of P can be read from attached graph. Until now, there is no good method for determining P different than with accompanying pictures. Research is under way to try to determine the value of P using calculation models that can simulate the water movement in the breakwater (OpenFOAM models).

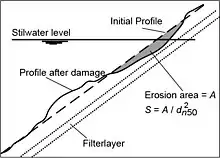
The value of the damage number S is defined as

S={\frac {A}{d_{n50}^{2}}}

[4]

where A is the area of the erosion area. Permissible values for S are:[2]

slope	Start of damage	Average damage, repair needed	Failure (core is exposed))
1:1,5	2	3-5	8
1:2	2	4-6	8
1:3	2	6-9	12
1:4	3	8-12	17
1:6	3	8-12	17

Definition of the damage area in the Van der Meer formula

References

Van der Meer, J.W. (1988). Rock slopes and gravel beaches under wave attack. TU Delft and Deltares. pp. 214 p.
CIRIA, CUR, CETMEF (2007). "5". The rock manual : the use of rock in hydraulic engineering. London: CIRIA C683. pp. 567–577. ISBN 9780860176831.{{cite book}}: CS1 maint: multiple names: authors list (link)
Coastal Engineering Manual EM 1110-2-1100, part VI,chapter 5. US Army Corps of Engineers. 2011. p. 74.
Broderick, L.L. (1983). "Riprap stability, a progress report". Proc. Coastal Structures ’83. American Society of Civil Engineering. pp. 320–330.

This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.

[vdMeer1988-1] Van der Meer, J.W. (1988). Rock slopes and gravel beaches under wave attack. TU Delft and Deltares. pp. 214 p.

[C683-2] CIRIA, CUR, CETMEF (2007). "5". The rock manual : the use of rock in hydraulic engineering. London: CIRIA C683. pp. 567–577. ISBN 9780860176831.{{cite book}}: CS1 maint: multiple names: authors list (link)

[CEM-3] Coastal Engineering Manual EM 1110-2-1100, part VI,chapter 5. US Army Corps of Engineers. 2011. p. 74.

[Broderick1983-4] Broderick, L.L. (1983). "Riprap stability, a progress report". Proc. Coastal Structures ’83. American Society of Civil Engineering. pp. 320–330.