Position angle
In astronomy, position angle (usually abbreviated PA) is the convention for measuring angles on the sky. The International Astronomical Union defines it as the angle measured relative to the north celestial pole (NCP), turning positive into the direction of the right ascension. In the standard (non-flipped) images, this is a counterclockwise measure relative to the axis into the direction of positive declination.
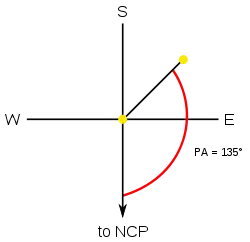
In the case of observed visual binary stars, it is defined as the angular offset of the secondary star from the primary relative to the north celestial pole.
As the example illustrates, if one were observing a hypothetical binary star with a PA of 135°, that means an imaginary line in the eyepiece drawn from the north celestial pole to the primary (P) would be offset from the secondary (S) such that the NCP-P-S angle would be 135°.
When graphing visual binaries, the NCP is, as in the illustration, normally drawn from the center point (origin) that is the Primary downward–that is, with north at bottom–and PA is measured counterclockwise. Also, the direction of the proper motion can, for example, be given by its position angle.
The definition of position angle is also applied to extended objects like galaxies, where it refers to the angle made by the major axis of the object with the NCP line.
Nautics
The concept of the position angle is inherited from nautical navigation on the oceans, where the optimum compass course is the course from a known position s to a target position t with minimum effort. Setting aside the influence of winds and ocean currents, the optimum course is the course of smallest distance between the two positions on the ocean surface. Computing the compass course is known as the inverse problem of geodesics.
This article considers only the abstraction of minimizing the distance between s and t traveling on the surface of a sphere with some radius R: In which direction angle p relative to North should the ship steer to reach the target position?
Global geocentric coordinate system
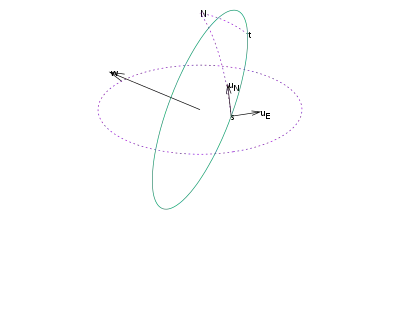
Detailed evaluation of the optimum direction is possible if the sea surface is approximated by a sphere surface. The standard computation places the ship at a geodetic latitude φs and geodetic longitude λs, where φ is considered positive if north of the equator, and where λ is considered positive if east of Greenwich. In the global coordinate system centered at the center of the sphere, the Cartesian components are
and the target position is
The North Pole is at
The minimum distance d is the distance along a great circle that runs through s and t. It is calculated in a plane that contains the sphere center and the great circle,
where θ is the angular distance of two points viewed from the center of the sphere, measured in radians. The cosine of the angle is calculated by the dot product of the two vectors
If the ship steers straight to the North Pole, the travel distance is
If a ship starts at t and swims straight to the North Pole, the travel distance is
Brief Derivation
The cosine formula of spherical trigonometry[1] yields for the angle p between the great circles through s that point to the North on one hand and to t on the other hand
The sine formula yields
Solving this for sin θs,t and insertion in the previous formula gives an expression for the tangent of the position angle,
Long Derivation
Because the brief derivation gives an angle between 0 and π which does not reveal the sign (west or east of north ?), a more explicit derivation is desirable which yields separately the sine and the cosine of p such that use of the correct branch of the inverse tangent allows to produce an angle in the full range -π≤p≤π.
The computation starts from a construction of the great circle between s and t. It lies in the plane that contains the sphere center, s and t and is constructed rotating s by the angle θs,t around an axis ω. The axis is perpendicular to the plane of the great circle and computed by the normalized vector cross product of the two positions:
A right-handed tilted coordinate system with the center at the center of the sphere is given by the following three axes: the axis s, the axis
and the axis ω. A position along the great circle is
The compass direction is given by inserting the two vectors s and s⊥ and computing the gradient of the vector with respect to θ at θ=0.
The angle p is given by splitting this direction along two orthogonal directions in the plane tangential to the sphere at the point s. The two directions are given by the partial derivatives of s with respect to φ and with respect to λ, normalized to unit length:
uN points north and uE points east at the position s. The position angle p projects s⊥ into these two directions,
- ,
where the positive sign means the positive position angles are defined to be north over east. The values of the cosine and sine of p are computed by multiplying this equation on both sides with the two unit vectors,
Instead of inserting the convoluted expression of s⊥, the evaluation may employ that the triple product is invariant under a circular shift of the arguments:
If atan2 is used to compute the value, one can reduce both expressions by division through cos φt and multiplication by sin θs,t, because these values are always positive and that operation does not change signs; then effectively
See also
Further reading
- Birney, D. Scott; Gonzalez, Guillermo; Oesper, David (2007). Observational Astronomy. Cambridge University Press. p. 75. ISBN 978-0-521-85370-5.
References
- Abramowitz, Milton; Stegun, Irene Ann, eds. (1983) [June 1964]. "Chapter 4.3.149". Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables. Applied Mathematics Series. Vol. 55 (Ninth reprint with additional corrections of tenth original printing with corrections (December 1972); first ed.). Washington D.C.; New York: United States Department of Commerce, National Bureau of Standards; Dover Publications. ISBN 978-0-486-61272-0. LCCN 64-60036. MR 0167642. LCCN 65-12253.