< Portal:Mathematics < Featured picture
Portal:Mathematics/Featured picture/2010 02
Picture of the month

Credit: Niabot
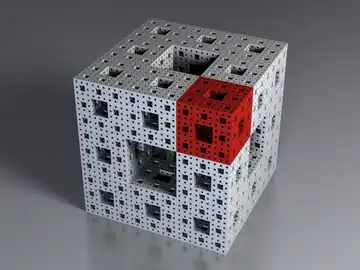
Menger sponge after four iterations.
The Menger sponge is a fractal curve. It is a universal curve, in that it has topological dimension one, and any other curve (more precisely: any compact metric space of topological dimension 1) is homeomorphic to some subset of it. It is sometimes called the Menger-Sierpinski sponge or the Sierpinski sponge. It is a three-dimensional extension of the Cantor set and Sierpinski carpet. It was first described by Karl Menger (1926) while exploring the concept of topological dimension.
| ...Archive | Read more... |
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.