Cantor tree surface
In dynamical systems, the Cantor tree is an infinite-genus surface homeomorphic to a sphere with a Cantor set removed. The blooming Cantor tree is a Cantor tree with an infinite number of handles added in such a way that every end is a limit of handles.
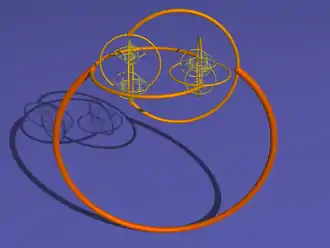
An Alexander horned sphere. Its non-singular points form a Cantor tree surface.
References
- Ghys, Étienne (1995), "Topologie des feuilles génériques", Annals of Mathematics, Second Series, 141 (2): 387–422, doi:10.2307/2118526, ISSN 0003-486X, MR 1324140
- Walczak, Paweł (2004), Dynamics of foliations, groups and pseudogroups, Instytut Matematyczny Polskiej Akademii Nauk. Monografie Matematyczne (New Series) [Mathematics Institute of the Polish Academy of Sciences. Mathematical Monographs (New Series)], vol. 64, Birkhäuser Verlag, ISBN 978-3-7643-7091-6, MR 2056374
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.