Geostationary Orbit

A satellite which orbits around the Earth at the same rate that the Earth turns is known as a synchronous orbit. Synchronous orbits can be of any inclination. If they are polar orbiting, the satellite will appear to be over the same spot at the same time every day.

Remote sensing satellites can be place in orbits that are synchronous with the Earth’s rotation over a longer period than a day, and thus will be able to view the entire ground surface over a number of orbits.

If a geosynchronous orbit is placed over the equator, something very interesting happens. The satellite will appear to stop moving in the sky. This is referred to as a geostationary orbit.

The gravitational acceleration of an object as a function of altitude is:

${\displaystyle a_{c}=\left({\frac {r_{e}}{r_{s}}}\right)^{2}g}$

where:

${\displaystyle r_{e}=}$ equatorial radius of the Earth (6378.388 km)

${\displaystyle r_{s}=}$ radius at the satellite position

${\displaystyle g=}$ Earth's gravitational constant (9.80665 m/sec²)

The centrifugal acceleration on a satellite is given by:

${\displaystyle a_{c}=r_{s}\omega ^{2}=r_{s}\left({\frac {2\pi }{T_{s}}}\right)^{2}}$

where:

${\displaystyle \omega =}$ angular velocity in radians/sec

${\displaystyle T_{s}=}$ time for 1 orbit (86400 seconds [24 hrs] for geostationary orbit)

For a stable orbit, the two forces associated with these accelerations must be equal

${\displaystyle \left({\frac {r_{e}}{r_{s}}}\right)^{2}g=r_{s}\left({\frac {2\pi }{T_{s}}}\right)^{2}}$

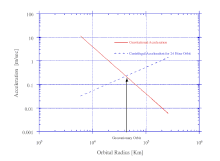
Solving for r_s we obtain:

${\displaystyle r_{s}={\sqrt[{3}]{\left({\frac {r_{e}T_{s}}{2\pi }}\right)^{2}g}}={\sqrt[{}]{\left({\frac {6378\ km\times 86400\ \sec }{2\pi }}\right)^{2}9.8065\ m/\sec ^{2}}}=42,254.22\ km}$

Therefor the height above the Earth is 42,254 km - 6378 km = 35,876 km or 22,292 miles.

The velocity of a satellite in a circular orbit is given by:

This works out to 3.073 km/Sec for a satellite in a geostationary orbit.

Molniya Orbit

The former USSR is not able to make great use of geostationary satellites, because of the northern latitude of the country. Consequently, communications satellites have required a slightly different approach. The term molniya means “flash of lightning”.

Sun Synchronous Orbit

Many satellites which require sunlight for imaging use sun-synchronous orbits. The sun-synchronous orbit provides a constant node-to-sun angle, and the satellite passage over a certain area occurs at the same time of the day.